Peutinger Table - a 19th century copy of a strip map printed in 1598

This is a 19th century print of a 12th century copy of a map used by the Roman Empire to accurately transport goods and instructions to the regions under its control from around 27AD to 576AD. It is an important treasure being the only known map of the Roman Empire and ranges from the coast of the United Kingdom, through to, purportedly, the coast of China.

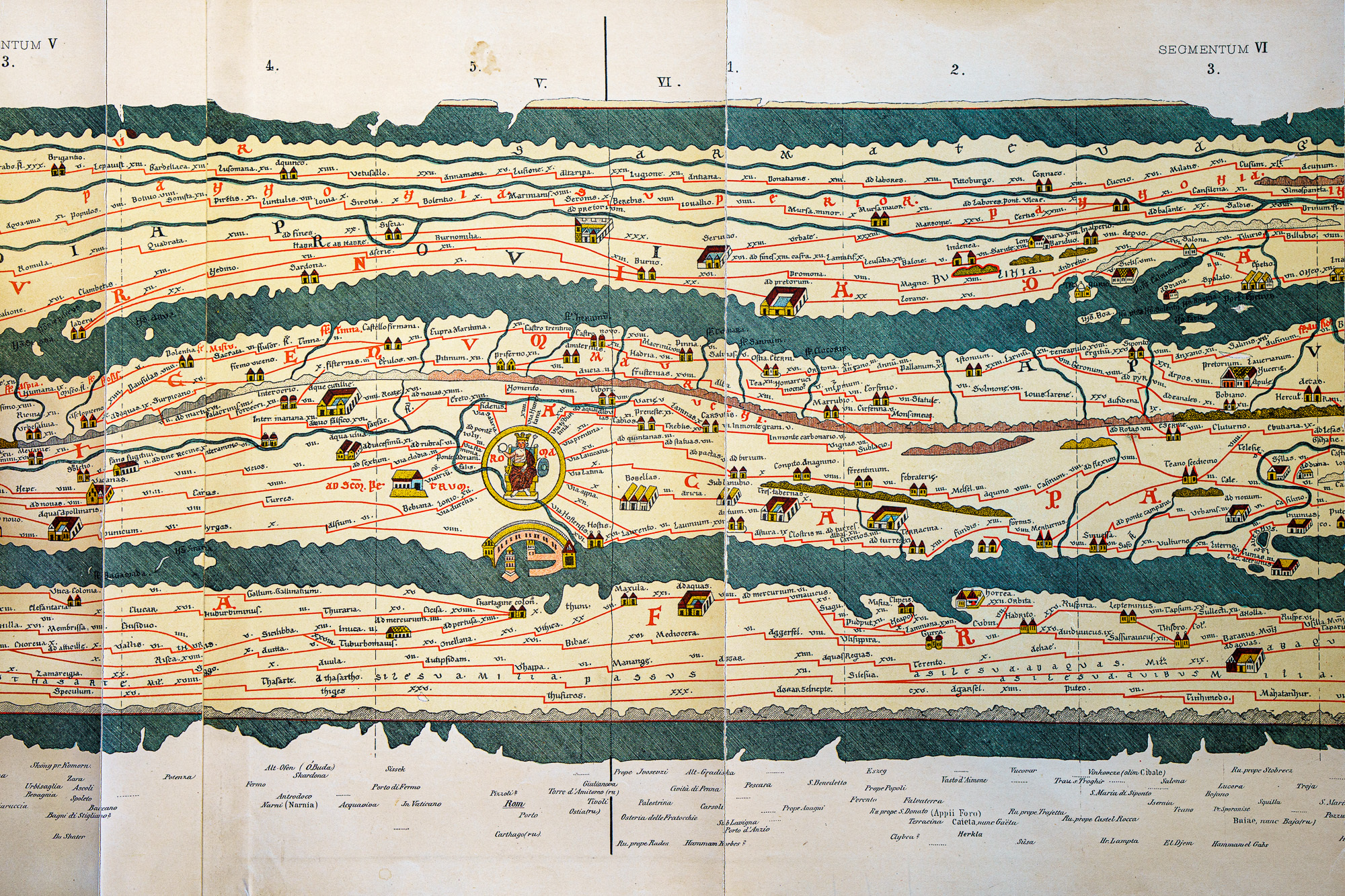
Maps of routes for specific purposes - military or trade - were produced by the Romans and revised, corrected and added to for the next 500 years. The Peutiger Table has no overall orientation and no consistent scale, resembling in this the 'strip maps' issued for specific journeys by the Royal Automobile Association.

Drawn in 1265 by a monk from Colmar - and now kept in the Austrian National Library - it is a medieval interpretation of the original Roman scroll which is no longer thought to exist. It is made up of 11 parchments scrolls and measures approximately 34 cm high by 6.74 m long when assembled. This document was discovered in 1494 by Konrad Meissel, alias Celtes, and given in 1507 to an Antiquarian of Augsburg, Konrad Peutinger, a Hapsburg court official. First printed in Antwerp in 1598 through the Plantin press and copied in the 19th century.

The oldest information on the map probably goes back to before 79 AD since Pompeii is indicated. Other temporal indications can be drawn from Jerusalem, which is named Aelia Capitolina, the name given in 132 AD, and from Constantinople (now Istanbul) the name being commonly used since the 5th century for Byzantium.

As expected all roads lead to Rome and the city is depicted at the centre of the 7m long scroll. The distances are in Roman Miles, which are 1,000 paces.

Map making (cartography) was usually done by taking prior copies, adding new knowledge, and then striking a new copy. Errors often crept into the maps, however this map is remarkably accurate for its time.

Located in the RGSSA Library, Mortlock Wing, the Peutinger strip map may be seen during opening hours.

RGSSA 207 gmbd, 4th century. It is located in the RGSSA Library, Mortlock Wing and may be viewed during opening hours.

The Peutinger Table, or Tabula Peutingeriana as it is also known, is a 19th century copy of a strip map printed in 1598 and originating from the 1st century.

Maps of routes for specific purposes — military or trade — were produced by the Romans, and this example is a 19th century print of a map dating back to the first century, revised, corrected, and added to for the next 900 years. It has no overall orientation and no consistent scale, resembling in this the 'strip maps' issued for specific journeys by the Royal Automobile Association. First issued by the Plantin press and printed in Antwerp in 1598, the printed map depicts the cities and roads from England (Kent and Norfolk) through to Türkiye , Iran, Pakistan, Afghanistan, India, Bangladesh and China and is in eleven sections. Parchment sections VII, IX, X and XI range from the Dardanelles through India to China. Here, the ancient world’s traditional span, from the Atlantic to India, is dramatically remoulded; lands and routes take pride of place, whereas seas are compressed.

Originally drawn in 1265 by a monk from Colmar and made up of 11 parchment scrolls measuring approximately 34 cm high by 6.74 m. long when assembled, this document was discovered in 1494 by Konrad Meissel, alias Celtes, and given in 1507 to an antiquarian of Augsburg, Konrad Peutinger.

The map is the only known iteneraria (an ancient Roman Road map) known to have survived from its use by curcus publicus, the courier service of the Roman Empire, which was created by the emperor Augustus (63BC – 14AD) to transport messages and tax revenues from one province to another. The absence of an Iberian Peninsula on the map suggests that the twelfth section may have been lost in antiquity. The map includes Roman icons and functional place symbols as well as the distances between each point.

Privacy

Peutinger Table - a 19th century copy of a strip map printed in 1598

RGSSA Catalogue and location

More about the Peutinger Table